
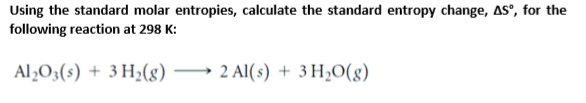
The purpose of this study is to assess the reliability of standard entropies compiled in those datasets. This is further compounded by the controversies surrounding the entropy values reported in established thermodynamic datasets for cements. 11–5.It is not straightforward to experimentally measure the standard molar entropy of cement hydrates or clinker minerals. Barin: Thermochemical Data on Pure Substances, 3rd ed. Martienssen, eds.: Springer Handbook of Materials Data, 2nd ed.
Arblaster: Selected Values of the Crystallographic Properties of the Elements, ASM, Materials Park, OH, 2018, pp. Ho, eds., Plenum Press, New York, 1975, pp. Saxena, ed., Springer, New York, 1992, pp. Guillermet: in Thermochemical Data: Advances in Physical Geochemistry, vol. Mohandas: Solid State Commun., 2002, vol. March: Electrons in Metals and Alloys, Academic, London, 1989, pp. Wang: Computational Thermodynamics of Materials, Cambridge University Press, Cambridge, 2016, pp. Fiquet: in Thermodynamic Data: Systematics and Estimation. Anderson: Equations of State of Solids for Geophysics and Ceramic Science, Oxford University Press, New York, 1995, pp. Spencer: Materials Thermochemistry, Sixth Revised Edition, Pergamon Press, Oxford, 1993, pp. Anderson: Theory of the Earth, Blackwell Scientific Publications, Boston, 1989, pp. Dittman: Heat and Thermodynamics: An Intermediate Textbook, 7th ed. Richet: The Physical Basis of Thermodynamics: With Applications to Chemistry, Springer, Heidelberg, 2001, pp. Grande: Chemical Thermodynamics of Materials: Macroscopic and Microscopic Aspects, Wiley, Chichester, 2004, pp. Cambridge University Press, Cambridge, 2017, pp. Anderson: Thermodynamics of Natural Systems: Theory and Applications in Geochemistry and Environmental Science, 3rd ed.

This power law correlation has been exploited to deduce the following outcome: (i) a simple approximation for the initial slope (d p/d T m) of p– T m melting curve, (ii) self-consistent correlation of entropy with specific heat and Debye temperature, (iii) estimation of entropy of metastable phases, and (iv) correlating dilute solution entropy with volume effects of alloying. A macroscopic power law relation for systematizing the standard entropy variation using a composite scaling parameter (MV 2/3/ T m) has been proposed, and its validity is demonstrated for both solid and liquid metals. The temperature and pressure dependencies of entropy have been discussed in terms of the complementing roles of S th and S el and simple approximations to these effects are suggested.

This argument is extended to establish a linear relationship between transformation entropy (Δ S tr) and transformation-induced volumetric strain (Δ V tr/ V). It is then argued that entropy variation among a group of alloy phases can be exclusively related to molar volume, only when both thermal pressure ( p th) and thermal entropy terms assume common values for all members. They are: (i) the pure thermal ( S th) contribution arising from the isochoric temperature dependence of Gibbs energy ( G T) and (ii) the elastic contribution ( S el) representing the dilatational volume effects. Two principal contributions to total nonconfiguration entropy ( S T) are outlined. This study presents a comprehensive analysis of the entropy of condensed phases, its temperature, pressure, and composition dependence on a macroscopic correlative platform.


 0 kommentar(er)
0 kommentar(er)
